Scientists Say: Imaginary Number

These weird numbers can multiply themselves into reality

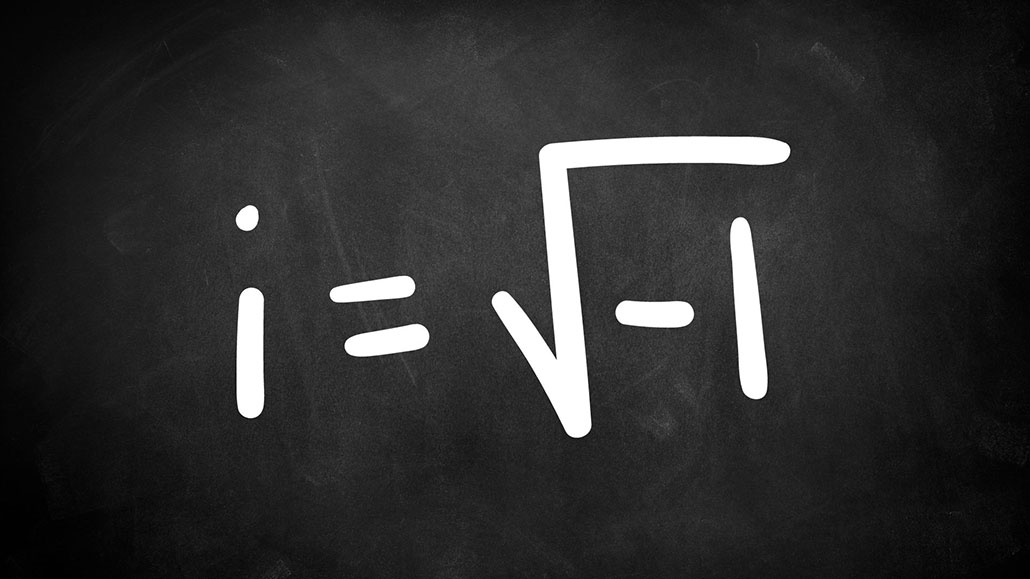
Imaginary numbers are represented by the symbol i. The symbol i represents the square root of -1.

StillFX/Getty Images Pro/CanvaPro; vitalintent/CanvaPro

October 2, 2023 at 6:30 am

Imaginary number (noun, “Ee-MAH-juh-neh-ree NUM-ber”)

An imaginary number is a kind of number that allows us to solve math problems that involve taking the square root of a negative number. They’re called “imaginary” because they don’t count or measure things, the way real numbers do.

All numbers you use to count or measure things in a typical day are known as real numbers. Simple numbers such as 1, 2 and 3 are real numbers. So are fractions and negative numbers. You can place real numbers on a number line.

But imaginary numbers are different. You can’t point out where an imaginary number would lie on a standard number line. Despite that, imaginary numbers aren’t make-believe. You can multiply, divide, add and subtract imaginary numbers just like real ones.

Imaginary numbers come into play when you need to take the square root of a negative number. Taking the square root of positive numbers is straightforward. A number’s square root is the number that, when multiplied by itself, gives the original number. For example, the square root of four is two. That’s because 2 × 2 = 4.

But taking the square root of a negative number is a little different. Consider -4, for instance. No number exists that you can multiply by itself to get -4. You might think that -2 multiplied by -2 should give you -4. But following the rules of mathematics, if you multiply two negative numbers, your result is a positive number. You cannot take the square root of a negative number.

To solve this problem, mathematicians hundreds of years ago defined a new type of number — imaginary numbers, represented by the symbol i. The symbol i represents the square root of negative 1, or -1. Mathematically, that looks like this:

i = √-1

Here’s the important part: if you multiply i by itself, you get -1.

i × i = -1

And -1 is a real number, not an imaginary one. So, equations using imaginary numbers can sometimes give non-imaginary answers.

We can use i to make an imaginary number answer for the square root of -4:

2i = √-4

That 2i is an imaginary number. It’s a placeholder, of sorts. But multiply it by itself and you get -4. That’s a real number.

Imaginary numbers make much of today’s technology possible. That’s because they play an important part in modeling the behavior of waves — such as light waves and sound waves. These kinds of waves transmit the information we receive through devices such as cell phones and other wireless technologies.